Topology is a large branch of theoretical (or “pure”) mathematics closely related to geometry. Like geometry, topology is a very visual subject. Instead of working with numbers, symbols and formulas, topologists and geometers study and compare objects in space. The difference between topology and geometry lies in the rules used to compare these objects. Loosely speaking, topologists have more relaxed rules than geometers. While geometers prefer rigid motions, topologists allow objects to be bent, stretched or twisted¾ as long as they are not broken or torn. Thus, a topologist considers a circle and a square to be the same, since one can be transformed into the other by bending and stretching¾as can be easily demonstrated with a piece of string or wire.

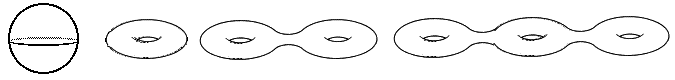
The field of topology is frequently broken into three sub-fields: general (or point-set) topology, algebraic topology, and geometric topology. Although I use some general and algebraic topology in my work, I am a geometric topologist. A particularly important type of object or “space” studied by geometric topologists is a manifold. A space is considered to be an n-dimensional manifold (or simply n-manifold) if, locally, it looks like Euclidean n-space. For example, a circle is a 1-manifold since a sufficiently nearsighted being living in a circle would not be able to distinguish his universe from a line (Euclidean 1-space). A more famous example is that persons living on the surface of a sufficiently large ball initially assume that their world is a Euclidean plane. Hence, the surface of a ball (known as a 2-sphere) is a 2-manifold. It and a few other 2-manifolds are pictured below.

 
How might a person living in one of the above worlds determine which world she is living in? An interesting problem for mathematicians and physicists is to determine which 3-dimensional or 4-dimensional manifold (depending on perspective) we are living in. Recent theories of physics suggest that our universe may actually be a manifold of dimension much higher than 4.

Most of my research involves the topology of manifolds. At this point in time, I am especially interested in “open” or “non-compact” manifolds. These are manifolds which are (in some sense) infinite in size. For example, the Euclidean plane¾ which extends infinitely outward¾ is non-compact; whereas the 2-sphere is compact. The extra flexibility permitted by non-compactness opens the door to exotic behavior “near infinity”. There are many examples of non-compact spaces that¾although they are constructed from simple finite pieces¾ have quite complicated structure at infinity. Through my research I hope to obtain a better understanding of these spaces.

Some other topics of my research are: metric geometry (a generalization of differential geometry), geometric group theory (a field in which topology is used to study algebraic objects known as groups), compactifications of manifolds and complexes, and spines of compact contractible manifolds. If you are interested in a more technical description of my research click here.